Rainbow
The rainbow is one of the most well-known optical effects related to weather and one of nature's most splendid masterpieces. A rainbow is an excellent demonstration of the dispersion of light and an evidence that visible light is composed of a spectrum of wavelengths, each associated with a distinct color.
Rainbows result from refraction of sunlight in falling water droplets plus reflection of the light from the back of the droplet.
Rainbows are more rare than might be thought. For example, in any one place in rainy England, there are fewer than ten bright ones in a year. That is due to the fact that the rainbow only occurs when there is sun- or moonshine, along with rainfall, in the direction of the rainbow. When you see a rainbow, the Sun is always behind, and the center of the circular arc of the rainbow is in the direction opposite to that of the Sun. The rain is in the direction of the rainbow.
Raindrops are never the tear shaped objects beloved of illustrators. Raindrops less than 2 mm in diameter are kept strongly spherical by surface tension forces. Larger drops are sometimes flattened by air resistance as they fall and they may even oscillate or wobble; even small departures from sphericity destroy a rainbow or possibly cause some odd effects.
If the Sun is not illuminating the raindrops, there will not be a rainbow. Also, when the Sun is too high in the sky (higher than about 42 degrees altitude), the rainbow is below the horizon and we will probably not see it.
Primary Rainbow
The most commonly seen rainbow, formed by light rays that undergo a single internal reflection in a drop of water, is called primary rainbow.
Its colours are produced by the two refractions as the rays enter and leave. Rays are deviated back towards the incoming sunlight to form a bow appearing opposite the Sun.
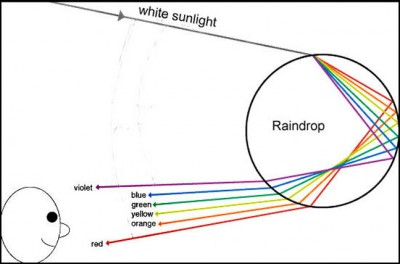Rays close to the drop centre are deviated almost 180º back on themselves. Rays further from the centre are deviated less and less until the deviation reaches a minimum (about 137.5º for deep red light). This is the "angle of minimum deviation" or "rainbow angle". The deviation increases once more as the entrance ray approaches the drop rim.
Rays cluster strongly around the rainbow angle, and so, the bow is at its brightest at that angle. Rays near the rainbow angle form the bow's bright outer edge.
Red light is refracted less than blue and its minimum deviation angle is less. Red is therefore on the outside of the primary bow.
Deviations are traditionally measured from the direction of the incoming sunlight. The deviation angle for red rays forming the edge of the primary bow is about 137.5º. The centre of a rainbow is directly opposite the Sun (a deflection angle of 180º). The radius of the red edge of the primary is therefore 180°-137.5° = 42.5º
The primary rainbow forms between about 38.72° and 42.86°. from the antisolar point.
Early morning and late afternoon are the best times to see rainbows because the Sun must not be too high. Rainbows are always opposite the Sun and their centres are below the horizon at the antisolar point. We don’t see a full circle because the Earth gets in the way. The lower the Sun is to the horizon, the more of the circle we see. At sunrise or sunset a rainbow's centre, the antisolar point, is on the horizon. The rainbow is half in the sky, a semicircle. As the Sun rises the bow's centre sinks. Eventually when the Sun is 42º high, only the tip of the bow is visible above the horizon.
The raindrops that contribute to a rainbow all lie on a cone with its apex at observer’s eye. So when you are looking at a rainbow, you are looking at the collected light from many raindrops, which for a fleeting moment, collectively produce a "cone of color" with its apex at your eye.
The distance of any particular drop from the observer’s eyes is not important; all of the drops on the cone’s surface scatter light rays back down to the observer’s eyes, and so the rainbow is not in any particular place in the sky.
If you move to the left or the right, your are looking at new raindrops, and hence, a new rainbow. If you are admiring a rainbow with a friend, you are both seeing different rainbows. The rainbow is different for different observers: that means each observer receives scattered light from different sets of raindrops and so, while different observers see a rainbow, it is not the same rainbow.
Secondary Bow
Light can be reflected more than once inside a raindrop. Rays escaping after two reflections make a secondary bow. The rays exit the drop at an angle of 50 degrees, rather than the 42 degrees for the red primary bow. Blue light emerges at an even larger angle of 53 degrees: this effect produces a secondary rainbow that has its colors reversed compared to the primary. The secondary has 43% of the total brightness of the primary but its surface brightness is lower than that because its light is spread over its greater angular extent. The primary and secondary are are concentric, sharing the antisolar point for a center.
A rainbow is not just a set of coloured rings. The sky inside is bright because raindrops direct light there too. The primary bow is a shining disk, brightening very strongly towards its rim.
Light rays undergoing a single reflection in raindrops form the primary rainbow or brighten the sky inside it. Rays reflected twice are deviated to form the secondary bow or brighten the sky outside.
So, the area between the primary and secondary bows is noticeably darken than the surrounding sky. Called “Alexander’s dark band”, it is named for the Greek sage Alexander of Aphrodisias, who described it in his chronicles in the early 3rd Century. This geometric band is an area devoid of rays which have passed through water drops. Light reflected from the surface of drops can reach this band, but those rays are relatively faint. In the case of a dewdrop spectrum, Alexander’s zone will appear very dark indeed because there are no competing sources of scattered light at this angle except for the surface reflection.
Supernumerary Bows
Look slightly inside a bright primary bow and sometimes you will see one or more predominantly green, pink and purple fringes: they are called “supernumerary bows”.
They occur when raindrops responsible for the main rainbow are small and much uniform in size, and their numbers and spacing can change from minute to minute.
The first explanation of supernumerary bows was provided by Thomas Young in 1804: they are an intimation of the limitations of geometric optics, for it is totally unable to explain them.
Supernumerary bows are a consequence of the wave-nature of light. The bands are caused by a phenomenon called light interference. Interference happens whenever waves of some sort in a medium interact with each other. At some places, the waves amplify each other, and at other places, they extinguish each other. Here, the waves are light waves, and the medium is air. Slightly different ray paths through a raindrop yield slightly different path lengths and slightly larger exit angles. As a result, there is constructive and destructive interference of each color in the spectrum as a function of ray exit angle, and a set of bows become visible inside the primary rainbow. Usually, there is some variation in the size of raindrops, and the supernumeraries are washed out altogether. There is almost always some washing out of colors, and the bows show much green and red in them, not the other colors in the spectrum.
Lunar Rainbow
The Moon is equally capable of producing rainbows, since all it takes to make a rainbow is a mass of water droplets and a light source.
A full Moon is bright enough to have its light refracted by raindrops, just as is the case for the Sun.
However, the lunar rainbow is usually so faint that you don't see colors, since we humans only see colors if the light is bright enough.
